Fourth grader Zaq asks the question, "If 6 times 6 equal 36 why doesn't 7 times 5 do the same?"

That's a great question Zaq. One of the easiest ways to understand multiplication is to think of it with pictures. Below, I show a picture of a 36 boxes in a great big square. The square has six rows and six columns.

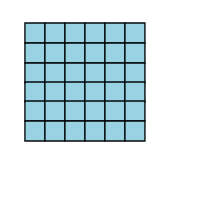
We say that 6 x 6 = 36 because if you have six rows, and each row has six objects in it, that's a total of 36 objects.

You can check it for yourself, by counting up all of those individual boxes in the picture. You'll find that there really are thirty-six of them.

But what about 7 x 5? If you had five rows, and there were seven objects in each row, would there be 36 objects? Well, let's find out. First, I'm going to take the row off the bottom, which will give us five rows instead of six.

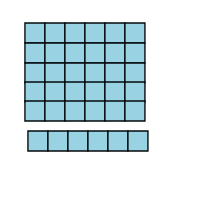
Now I'm going to take that row and rotate it around so it'll fit on the side of the picture.

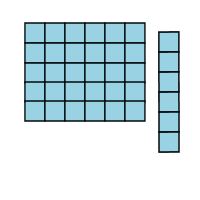
Uh oh...you see what's going to happen, right? We're going to have an extra block! There are two many rows in that group of boxes, so there'll be one poor little box, all stuck by himself, that doesn't fit into the picture.

And that one block shouldn't come as a surprise to you, because you know what 7 x 5 is - it's 35, which is one less than 6 x 6! And sure enough, that "one less" is the reason why we have a leftover box at the end.

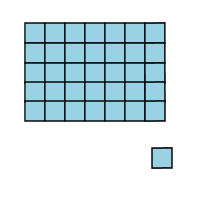
You might also be interested to know that that "one less" idea also works whenever you multiply a number by itself: 7 x 7 is 49, but 6 x 8 is one less than that. 10 x 10 is 100, but 9 x 11 is one less than that. 12 x 12 is 144, but 11 x 13 = 143. It's easy to see why that is; whenever you try to take a perfect square and turn a row into a column like we did above, you'll end up with one extra block!

And someday, you'll take an alegbra class, and you'll learn a different way of explaining why that works, but for now, hopefully you'll find the picture a good way of understanding it. Thanks for asking!