Yesterday we received the following question: "What is estimating?"

This is a fairly broad question, but it's worth answering.  I'll explain briefly what it is, and then after that I'll talk about why it's important to be able to estimate.

The following is a screen shot from our game "Guess It!" in the Junior section of the site.

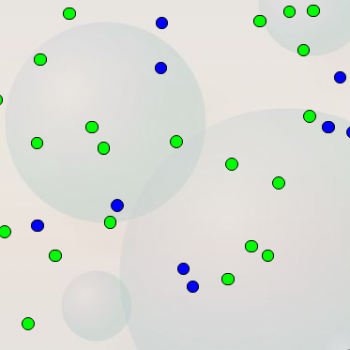
When you are playing "Guess It," the site might ask you a question like "Are there more green dots or blue dots?" You will automatically say, "There are more green than blue." But how do you know? Did you stop to count all the green dots, and then count all the blue dots? Hopefully you didn't! Hopefully you were able to quickly scan the image and recognize that there are more green, without taking the time to count them. That's estimating.

The site might ask you "Is the number of green dots closer to 10 or 50?" Do you need to actually count the green dots in order to answer the question? No!  You've probably got a good sense for what a group of ten dots would look like, and it's fairly obvious that the image doesn't contain 5 groups like that. So you would say "It's closer to 10 than 50!" That's estimating.

Estimating is when we don't need to have an exact answer; we just want to be "in the ballpark."

Why Is It Important?

Suppose you had $50.00 in your wallet, and you went grocery shopping. You don't have a calculator with you, and you don't want to add all your purchase prices by hand, but you want to be sure you don't get to the cashier with a cart full of items that add to more than $50. Your items cost $7.96, $3.20, $13.84, and $4.42. You can do some estimating like this: "I'll round all my items up to the next multiple of five so I can add them easily. If the sum is less than 50, then I know I'm okay. $10, $5, $15, $5. 10 + 5 + 15 + 5 = 35, so I'm okay!"

Estimation is also a great way of checking to see if your conclusions are reasonable. If I'm trying to calculate how long it will take an object to fall to the ground from the roof of my house (yeah, I calculate silly stuff like that, because I'm a Physics teacher!), I can mentally picture it falling, and estimate: "Well, it certainly won't take more than 5 seconds!" and so if I do my calculations and come up with an answer of 35 seconds, I know something must be wrong!

I do a lot of estimating while I'm teaching. Using the "falling to the ground" example above, I know that the acceleration due to gravity is 9.8 m/s², which is not a nice number to work with if you don't have a calculator. But it's really close to 10, so if I'm doing a freefall problem on the board, and don't want to pick up a calculator, I'll do an estimation by replacing 9.8 with 10, and work out an approximate answer in my head. Then, when my students start telling me the numbers they came up with, I'll ask myself, "Is that reasonably close to my answer?" If it is, I'll feel confident that they got the answer correct.

In general, I find that my students who are good at estimation do much better than the ones who do not, because they are quicker to recognize whether their answers even make sense! That's not just a good thing in school; it's a skill that will transfer to your job and your every-day life.