Krish asks, "In your base 11 Vortex Math, what brought you to begin with the number 2 rather than 1?"

For those who don't know what this question is in reference to, you'll want to visit our Vortex Based Mathematics page, where we explore (for a little bit of fun and enlightenment) what Vortex Based Math would have looked like if Marko Rodin was the proud owner of eleven fingers instead of just ten. It's a bit tongue-in-cheek, but it's a way of helping people understand the fundamental concept that Marko missed out on: bases.

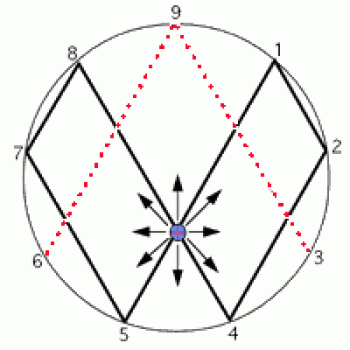
In Marko's base ten diagram (which he and his followers have been known to refer to as an equation, even though picture  and equation are not even remotely similar), Marko uses a doubling process to create a "loop" of numbers.

The numbers 1, 2, 4, 8, 7, and 5 each map to the next number in the sequence, with the number 5 mapping back to the number 1.

This produces an approximate infinity symbol, which, to Marko's way of thinking implies that this is going to result in an infinite supply of energy for everyone.

So my imaginary Seussian friend from Wunfishia, whose name is Marko-11 (because he has eleven fingers) did the whole process in base eleven. And his diagram looks like the one over on the right.

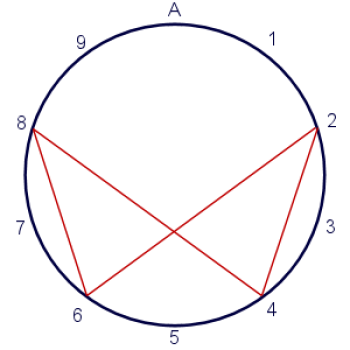
Notice that Marko-11 skipped over the number 1, and started his loop with the number 2. This is the source of Krish's question: "what brought you to begin with the number 2 rather than 1?"

The answer to that question might surprise you: I didn't start with the number 2; I started with the number 1! This was when I was doing the math for myself before I started writing up the page. 1 maps to 2, 2 to 4, and so forth. I was actually a little surprised when the mapping from 6 went back to 2 instead of to 1.

That was when I discovered "feeders" - numbers that aren't part of the loop, but feed into it. If you look at the picture below, you'll see that in base eleven, the vortex diagram has four feeders - four numbers that are not part of the loop, but feed into it.

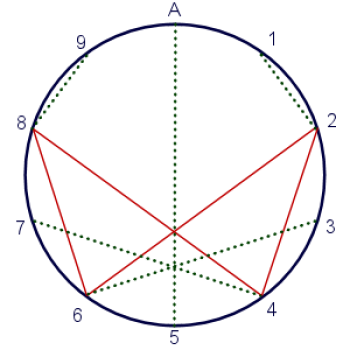
Once I understood that, I realized that it would be simpler to start with 2 when I was explaining VBM-11, and then show the "feeders" once I established the loop. The reason for doing that was purely pedagogical; I thought it would be easier for people to understand if I fully explained one concept before moving on to the next.

So let's wrap up with one more interesting question (which I have not yet explored):

In some bases, the loop starts with the number 1, in some bases it starts with the number 2, and in some cases it even starts with 3 or 4 (here's an example that starts with 4: Vortex-29). The question is: what is the pattern? Someday I may explore that question mathematically, but in the meantime, if you go to our Vortex-n-k simulator page, the website will do all the doubling and looping math for you, so you can quickly scroll through various vortex diagrams and see which loops start with 1, and which ones don't.

Have fun!
Professor Puzzler